Question

Prove that the curve a(t) = (cost, sin 2t, cos 2t) is regular on R and that it self-intersects at (1,0,1). Check the self-intersection part by using algebra and also by using Geofte

Answer 1

The function a (t) is a vector function composed of the component functions
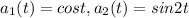`a_ {1} (t) = cost, a_ {2} (t) = sin2t` and
`a_ {3} (t) = cos2t`. How
`a_ {1} (t), a_ {2} (t), a_ {3} (t)` are infinitely derivable functions in R, so they are regular functions in R.

Now, for
`t = 0`, you have to
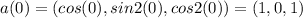`a (0) = (cos (0), sin2 (0), cos2 (0)) = (1, 0, 1)`. How the functions
`a_ {1} (t), a_ {2} (t), a_ {3} (t)` are periodic functions with period
`2 \pi,` the vector function
`a (t)` will take the same point
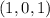`(1, 0 , 1)` at
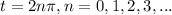`t = 2n\pi, n = 0, 1, 2, 3, ...` then the vector function is auto-intercepted

Explanation: