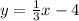Answer:
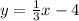
Explanation:
Given equation :-
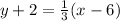
• Slope intercept form is
y = mx + c
where , m is slope of the straight line
and c is y intercept of the line !
Solving and simplifying the given equation ,
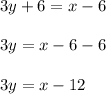
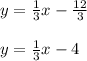
So, m = 1/3 and c = ( -4 )
hence , the required slope intercept form of the equation is