Question

While standing on a bridge 15.0 m above the ground, you drop a stone from rest. When the stone has fallen 3.20 m, you throw a second stone straight down. What initial velocity must you give to the second stone if they are both to reach the ground at the same instant?

Answer 1

11.35 m/s

Step-by-step explanation:

Let the first ball takes time t to reach the ground.

Use second equation of motion

`s=ut + 0.5at^(2)`

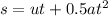
Here, s = 15 m, a = 9.8 m/s^2, u = 0 m/s

By substituting the values

15 = 0 + 0.5 x 9.8 x t^2

t = 1.75 second

Now time taken by the first ball to reach 3.20 m is t'

Use second equation of motion

`s=ut + 0.5at^(2)`

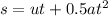
Here, s = 3.2 m, a = 9.8 m/s^2, u = 0 m/s

By substituting the values

3.2 = 0 + 0.5 x 9.8 x t'^2

t' = 0.81 s

Thus, the time for the second ball to reach the ground is t - t' = 1.75 - 0.81 = 0.94 s

For second ball:

Use second equation of motion

`s=ut + 0.5at^(2)`

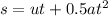
Here, s = 15 m, a = 9.8 m/s^2, u = ?, t = 0.94 s

By substituting the values

15 = u x 0.94 + 0.5 x 9.8 x 0.94 x 0.94

u = 11.35 m/s

Thus, the second ball has the initial velocity of 11.35 m/s.

Answer 2

`v = 11.34 m/s`

Step-by-step explanation:

After 3.20 m of free fall the time taken by the stone is given as

`d = (1)/(2)gt^2`

so here we will have

`3.20 = (1)/(2)(9.8)(t^2)`

`t = 0.81 s`

so speed of the stone is given as

`v_f = 0 + 9.81(0.81)`

`v_f = 7.93 m/s`

now when other stone is thrown downwards

then the relative speed of the two stones is given as

`v_r = v_1 - v_2`

`v_r = v - 7.93`

now the time taken by the stone to drop down by 15 m

`15 = (1)/(2)(9.81) t^2`

`t = 1.75 s`

so the time remaining to catch the two stones is given as

`\Delta t = 1.75 - 0.81 = 0.94 s`

so we have

`(v - 7.93)* 0.94 = 3.20`

`v = 11.34 m/s`