Question

A hockey player is standing on his skates on a frozen pond when an opposing player, moving with a uniform speed of 2.0 m/s, skates by with the puck. After 2.00 s, the first player makes up his mind to chase his opponent. If he accelerates uniformly at 0.24 m/s2, determine each of the following. (a) How long does it take him to catch his opponent? (Assume the player with the puck remains in motion at constant speed.)

Answer 1

18.47 s

Step-by-step explanation:

Given:

Speed of the opponent, u₁ = 2 m/s

Time after which player starts = 2 seconds

Thus,

distance covered (s₁) by the opponent before the player starts = u₁ × 2

= 2 × 2 = 4 m

let 't' seconds be the time taken by the player to catch the opponent

now,

the total distance covered by the player will be

= 4 m + distance traveled by the opponent in the time t

= 4 + ( 2 × t ) m

also,

From Newton's equation of motion the distance covered by the player

`s_2=ut+(1)/(2)at^2`

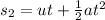
where,

s is the distance

u is the initial speed = 0 m/s as starting from rest

a is the acceleration = 0.24 m/s²

t is the time

on substituting the respective values, we get

`s_2=0* t+(1)/(2)*0.24* t^2`

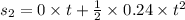
also,

s₁ = s₂

thus,

`4 + 2* t=0* t+(1)/(2)*0.24* t^2`

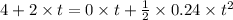
or

4 + 2t = 0.12t²

or

0.12t² - 2t - 4 = 0

on solving the quadratic equation, we get

t = 18.47 s

[negative value of the roots is ignored as time cannot be negative]