Answer:
a) The probability that the chosen ball is blue is 56.84%
b) If the chosen ball is blue, there is a 35.19 probability that it came from the first urn.
Explanation:
a) What is the probability (as a %) that the chosen ball is blue?
First the urn is chosen, then the ball. There can be a blue ball from urn 1 and from urn 2.
-The probability of a blue ball being chosen from urn 1 is:
40%(8 blue balls among 20 in urn 1, 8 of 20 is 40%) of 50%(the chance of urn 1 being chosen is 50%)
So
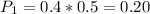
-The probability of a blue ball being chosen from urn 2 is:
73.68%(14 blue balls among 20 in urn 2, 14 of 19 is 73.68%) of 50%(the chance of urn 2 being chosen is 50%)
So
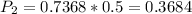
The probability that the chosen ball is blue is
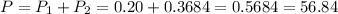 %.
%.
b) If the chosen ball is blue, what is the probability (as a %) that it came from the first urn?
This item can be formulated as the following problem:
What is the probability of B happening, knowing that A has happened.
It can be calculated by the following formula
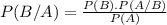
Where P(B) is the probability of B happening, P(A/B) is the probability of A happening knowing that B happened and P(A) is the probability of A happening.
In this item, A(what has happened) is the ball being blue. In item a), we found that P(A) = 0.5684.
B is the blue ball coming from the first urn. P(B), the probability of the first urn being chosen, is 50% = 0.5.
P(A/B), the probability of the blue ball being chosen from the first urn is 40% = 0.4.
So:
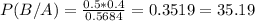
If the chosen ball is blue, there is a 35.19 probability that it came from the first urn.