Question

A railroad trestle spans a gorge 80 feet wide and connects two cliffs at heights of 112 and 172 feet above the bottom of the gorge. A train is crossing this gorge from the higher cliff to the lower. When the front of the train has traveled $\frac{3}{4}$ of the trestle's length, how many feet is it above the bottom of the gorge?

Answer 1

172 - 112 = 60 feetSince it is a straight line of constant slope, the train will have dropped 3/4 * 60 = 45 ft from the 172 ft side172-45 = 127ft above the floor of the gorge.

Answer 2

There are 157 ft. above the bottom of the gorge

Step-by-step explanation:

Cliff 1 (h) = 172ft.

Cliff 2(h) = 112 ft

Height difference between the cliffs: 172ft. -112 ft. = 60 ft

Width of the gorge = 80 ft

In this problem a right triangle is formed where the hypotenuse (T) is represented by the trestle's length, the width of the gorge is one of the legs and the leg opposite the angle (Ф) is represented by the difference in height between the cliffs.

T=
`√(L_1^2 + L_2^2 ) =√(80^2 + 60^2) =√(10,000) =100 ft`

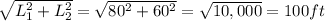
3/4 T = 3/4(100) = 75ft.

Calculating the height of the train from the lowest cliff, we will use the proportion of the sine of the angle that forms the trestle with the horizontal.

sin(Ф)=
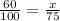
`(60)/(100) = (x)/(75)`

x=
`(60*75)/(100)=45ft`

In order to know the height from the bottom of the cliff, we must add its height to this value.

h= 45ft. + 112ft. = 157 ft.