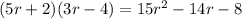Answer:
Explanation:
The given expression is
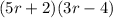
To find the product of this expression, we have to use the distributive property, which is developed as follows
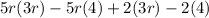
As you can observe, this property consists in "distributing" the first factor to the second factor. Now, we solve each product and then like terms.
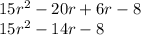
Therefore, the product is