Question

Use the echelon method to solve the following system of two equations in two unknowns. Check your answer. x+y=17 amd 9x-y=73

Answer 1

y = 8

x = 9

Explanation:

given equation,

x + y = 17 ........(1)

9 x - y = 73...........(2)

writing in matrix form

A X = B

`\begin{bmatrix}1 &1 \\ 9 &-1 \end{bmatrix} \begin{bmatrix}x\\ y\end{bmatrix}=\begin{bmatrix}17\\73 \end{bmatrix}`

using augamented matrix A|B

`\begin{bmatrix}1 &1& 17\\ 9 & -1 & 73\end{bmatrix}`

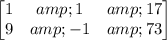
using row transformation

R₂ = R₂ - 9 R₁

`\begin{bmatrix}1 &1& 17\\ 0 & -10 & -80\end{bmatrix}`

now,

`\begin{bmatrix}1 &1 \\ 0 &-10 \end{bmatrix} \begin{bmatrix}x\\ y\end{bmatrix}=\begin{bmatrix}17\\-80 \end{bmatrix}`

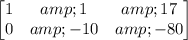
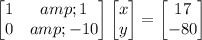
now

-10 y = -80

y = 8

and

x + y = 17

x = 17 -8

x =9