Answer: The heat required by iron is 1307.11 Joules or 312.4 Cal.
Step-by-step explanation:
To calculate the amount of heat released or absorbed, we use the equation:
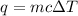
where,
q = heat absorbed
m = mass of ice = 28.4 g
c = specific heat capacity of ice = 0.5Cal/g.°C
 = change in temperature =
= change in temperature =

Putting values in above equation, we get:
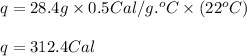
Converting the value from calories to joules, we use the conversion factor:
1 J = 0.239 Cal
So,

Hence, the heat required by ice is 1307.11 Joules or 312.4 Cal.