Step-by-step explanation:
As there are 1000 mL present in 1 L. So, 393 ml will be equal to 0.393 L. And, concentration of
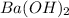 present is 0.171 M.
present is 0.171 M.
As molarity is the number of moles present in liter of solution.
Molarity =
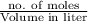
Therefore, putting the given values to calculate the number of moles as follows.
Molarity =
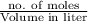
0.171 M =
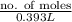
no. of moles =
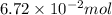
Thus, we can conclude that the number of moles of
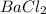 formed are
formed are
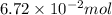 .
.