Step-by-step explanation:
a) Using Beer-Lambert's law :
Formula used :

where,
A = absorbance of solution = 0.22
C = concentration of solution =
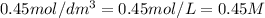

l = length of the cell = 3.0 cm
 = molar absorptivity of this solution = ?
= molar absorptivity of this solution = ?
Now put all the given values in the above formula, we get the molar absorptivity of this solution.
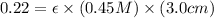
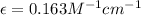
Therefore, the molar absorptivity of this solution is,

b)
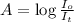
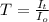
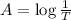
A = 2 × 0.22 =0.44
 = Intensities of Incident light and transmitted light respectively
= Intensities of Incident light and transmitted light respectively
T = Transmittance

T = 0.3630
c)
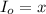

Thickness of cell = l' =?

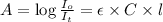
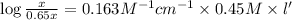
l' = 1.53 cm
d) No, we cannot calculate the absorbance at 590 nm from the given data. This is because absorbance at this wavelength can be observe experimentally.