Question

A concave mirror has a focal length of 13.5 cm. This mirror forms an image located 37.5 cm in front of the mirror. Find the magnification produced by the mirror.

Answer 1

Step-by-step explanation:

It is given that,

Focal length of the concave mirror, f = -13.5 cm

Image distance, v = -37.5 cm (in front of mirror)

Let u is the object distance. It can be calculated using the mirror's formula as :

`(1)/(v)+(1)/(u)=(1)/(f)`

`(1)/(u)=(1)/(f)-(1)/(v)`

`(1)/(u)=(1)/((-13.5))-(1)/((-37.5))`

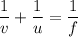
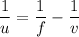
u = -21.09 cm

The magnification of the mirror is given by :

`m=(-v)/(u)`

`m=(-(-37.5))/((-21.09))`

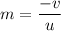
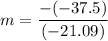
m = -1.77

So, the magnification produced by the mirror is (-1.77). Hence, this is the required solution.