Question

A 2.55-m-long rod, as measured in its rest frame, speeds by you longitudinally at 6.11Ã10^7. You measure its length as it passes. By how many millimeters do you determine the rod has contracted?

Answer 1

The length contracts by 54 mm.

Step-by-step explanation:

According to theory of special relativity the contraction in the length of an object travelling at a speed 'v' is given by

`L=L_(o)\sqrt{1-(v^(2))/(c^(2))}`

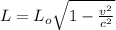
where

L = contracted length of the object

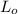`L_(o)` = original length of object

v = speed of the object

c = speed of light

Applying values we get

`L=2.55* \sqrt{1-((6.11* 10^(7))^(2))/((3* 10^(8))^(2))}`

`L=2.496m`

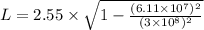
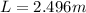
Thus the change in length equals
`\Delta L=2.55-2.496\\\\\therefore \Delta L=0.054m=54mm`