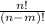Question

How many one-to-one functions are there from a set with m elements to a set with n elements, where msn?

Answer 1

Explanation:

Given that there are two sets one with m elements and other with n elements and m<n.

If there is a one to one correspondence from first set to second set, each element in first set will have a unique image, different from the images of other elements inthe set.

I element can be mapped in n ways second in n-1 ways .... and last one in n-m+1 ways.

Hence no of ways = nPm

=
`(n!)/((n-m)!)`