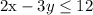The points (0,-4) and (3,-2) are on the boundary line.
Find the slope
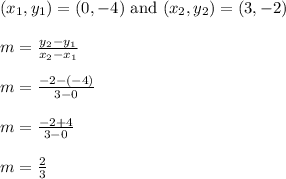
The y intercept is b = -4 since this is where the graph crosses the y axis.
Therefore,
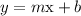 will turn into
will turn into
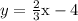 which is in slope intercept form.
which is in slope intercept form.
--------------
From here, we convert to standard form Ax+By = C
Multiply both sides by the LCD 3 and then rearrange terms so that the x and y terms are on the same side

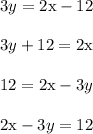
The equation of the boundary line, in standard form, is 2x-3y = 12
--------------
The last thing to do is to change the equal sign to one of the four inequality signs. The question is: which one?
Well we know there's an "or equal to" as part of the inequality sign due to the solid boundary line. If the boundary line were dotted or dashed, then we wouldn't have "or equal to".
So we'll go for either
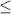 or
or
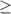
Let's try out a test point (0,0). This means we plug in x = 0 and y = 0
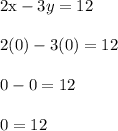
Clearly the last statement is false, but we can fix things by replacing each equal sign with a
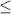 sign
sign
So,
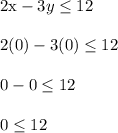
The last statement is true, which domino effects toward making the first statement true when (x,y) = (0,0). Note: if the boundary line went through the origin, then pick another point such as (0,1).
This effectively shows the shaded region involves the origin. Therefore we shade above the solid boundary line as shown in the given diagram.
This fully confirms why the answer is