1 Answer

Mir Rahed Uddin · Answer 1 · 2020-07-30T01:12:03+0000

Answer:

See attachment.

Explanation:

The given inequality is
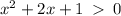
$x^2+2x+1\:>\:0$

We can rewrite this inequality as
$(x+1)^2\:>\:0$

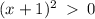
Or

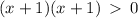
$(x+1)(x+1)\:>\:0$

The solution to this inequality is {x<-1,x>-1}

The solution is all real numbers except -1

We can rewrite this as x≠-1

The graph is shown in the attachment

Please log in or register to add a comment.