Question

Suppose that the function f has domain all real numbers. Determine whether each function can be classified as even or odd. Explain.

a) g(x)= [f(x) + f(-x)]/2
b) h(x)= [f(x) – f(-x)]/2

Answer 1

a.Even

b.Odd

Explanation:

We are given that function f has domain all real numbers .

We have to find that given function is even or odd

a.
`g(x)=(f(x)+f(-x))/(2)`

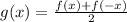
We know that if function is even then f(x)=f(-x) and if function is odd then
`f(x)\\eq f(-x)`

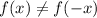
Replace x by -x then we get

`g(-x)=(f(-x)+f(x))/(2)=g(x)`

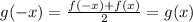
Therefore, function is even .

b.
`h(x)=(f(x)-f(-x))/(2)`

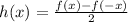
Replace x by -x

Then we get
`h(x)=(f(-x)-f(x))/(2)=-(f(x)-f(-x))/(2)`

`h(x)\\eq h(-x)`

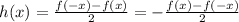
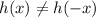
Hence, function is odd.