Answer: 3,893,845.918 Ping-Pong balls
Explanation:
The volume of an average room is:
 (1)
(1)
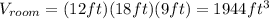 (2)
(2)
Now let’s transform this
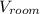 to units of
to units of
 , knowing
, knowing
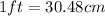 :
:
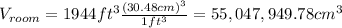 (3)
(3)
On the other hand, we have Ping-Pong balls with a radius
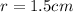 , and their volume is given by:
, and their volume is given by:
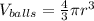 (4)
(4)
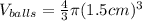 (5)
(5)
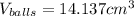 (6)
(6)
Now, the number
 of Ping-Pong balls that can be packed into the room is:
of Ping-Pong balls that can be packed into the room is:
 (7)
(7)
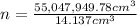 (8)
(8)
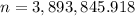 This is the number of Ping-Pong balls that can be packed into an average size room
This is the number of Ping-Pong balls that can be packed into an average size room