Question

The total energy of a particle: 1) is not related to its relativistic momentum.

2) increases with increasing relativistic momentum.
3) decreases with increasing relativistic momentum.
4) is a constant.
5) behaves differently for different particles.

Answer 1

(2) Increases with increasing the relativistic momentum

Step-by-step explanation:

We know that kinetic energy of a moving body of mass m and velocity v is given by
`KE=(1)/(2)mv^2`

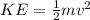
Multiplying both side by mass
`m* KE=(1)/(2)m* mv^2`

`2mKE=(mv)^2`

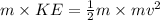
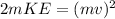
We know that momentum p =mv

So
`2mKE=p^2`

`p=√(2mKE)`

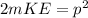
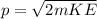
So from the relation we can see that kinetic energy is proportional to the momentum so if we increase the momentum then kinetic energy also increases