Answer:
99.67 cm to the left of second lens
Step-by-step explanation:
For the first lens :
 = Distance of the object = 18 cm
= Distance of the object = 18 cm
 = focal length of the lens = 9 cm
= focal length of the lens = 9 cm
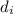 = Distance of the image
= Distance of the image
Using the lens equation
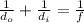
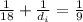
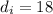 cm
cm
 = distance between the two lenses = 29.5 cm
= distance between the two lenses = 29.5 cm
For the second lens, the image formed by the first lens acts as the object
For the second lens :
 = Distance of the object =
= Distance of the object =
 =
=
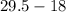 =
=
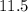 cm
cm
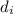 = Distance of the image
= Distance of the image
 = focal length of the lens = 13 cm
= focal length of the lens = 13 cm
Using the lens equation
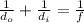
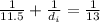
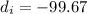 cm
cm
The image is located 99.67 cm to the left of the second lens