Question

Which graph represents this system?
2x-5y=-5
Y=2/5x+1

Answer 1

both equations line up

Answer 2

The given system is formed these equations

`2x-5y=-5`

`y=(2)/(5)x+1`

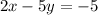
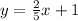
If you pay careful attention to this system, you would see that both equations represent the same thing, but they are just written in different ways. To demonstrate this, let's isolate
`y` in the first equation

`2x-5y=-5\\-5y=-5-2x\\y=(-5-2x)/(-5)\\y=1+(2)/(5)x`

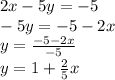
Which is the same than
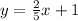`y=(2)/(5)x+1`. But, what does it mean this?

It means that the graph must show one line over the other one, or you can see it as the same single line, because both equation are just the same. This also means that the system has infinite solutions.

The image attached shows the behaviour described.