Question

Which statements correctly describe the graph of the function f(x)=x^3-4x^2-3x+18? select three options.​

Answer 1

2,3,5

Explanation:

Answer 2

The function has a double root

As x increases from negative infinity to positive infinity, the y-values increase, decrease and then increase

The domain and range of the function are the set of real numbers

Explanation:

we have

`f(x)=x^(3)-4x^(2)-3x+18`

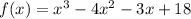
using a graphing tool

see the attached figure

we know that

1) The y-intercept of the function f(x) (value of y when the value of x is equal to zero) is

For x=0

f(0)=18

so

The y-intercept is the point (0,18)

2) The roots of the function (or x-intercepts) are

x=-2 -----> with a multiplicity of 1

x=3 -----> with a multiplicity of 2

so

The x-intercepts are (-2,0) and (3,0)

3) As x increases from negative infinity to positive infinity, the y-values increase, decrease and then increase

4) As x approaches negative infinity , y approaches negative infinity

5) As x approaches positive infinity , y approaches positive infinity

6) The domain and range of the function are the set of real numbers