Question

Ball A is dropped from the top of a building of height h at the same instant that ball B is thrown vertically upward from the ground. When the balls collide, they are moving in opposite directions, and the speed of A is twice the speed of B. At what height does the collision occur?

Answer 1

(2/3) times height collision occur

Step-by-step explanation:

for ball A

from the kinematic equation

the distance of ball A is

`x_A = v_0 t + (1)/(2) at^2`

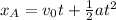
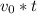`v_0* t`= velocity ( time ) = distance

since ball is at height, the above equation changes as

`x_A = H - (1)/(2) gt^2`

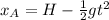
for ball B

`xB = v0 t - (1)/(2) gt^2`

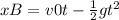
the condition of collision is

xA = xB

`vA = - 2vB` (given)

from the kinematic equation

the speed of the ball A is

`v_A = u- gt`

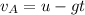
since initial speed of the ball A is zero

, so

`v_A = -gt`

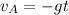
the speed of the ball B is

`v_B = v_0 - gt`

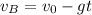
since
`v_A = - 2v_B`

`-gt = -2 ( v_0 - gt)`

`-gt = -2 v_0 +2gt`

`3gt =2 v_0`

`t = (2v_0)/(3g)`

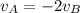
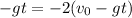
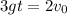
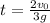
since
`x_A = x_B`

`H -  (1)/(2) gt^2= v_0 t - (1)/(2) gt^2`

`H = v_0 t`

`= v_0 (2v_0/3g)`

`= (2 v_0^2)/( 3g)`

`x_A = 2 ((v_0^2)/( 3g))- (1)/(2) gt^2`

`= 4 (v_0^2)/(9g) = (2/3) H`

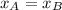
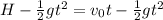
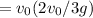
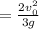
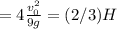
so, (2/3) times height collision occur