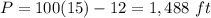Question

The area of a square in square feet is

represented by 625z^2 − 150z + 9. Find an
expression for the perimeter of the
square. Then find the perimeter when
z = 15 ft.

Answer 1

Part 1) The expression for the perimeter is
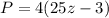`P=4(25z-3)` or
`P=100z-12`

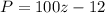
Part 2) The perimeter when z = 15 ft. is
`P=1,488\ ft`

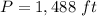
Explanation:

Part 1)

we have

`625z^(2)-150z+9`

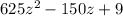
Find the roots of the quadratic equation

Equate the equation to zero

`625z^(2)-150z+9=0`

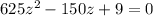
Complete the square

Group terms that contain the same variable, and move the constant to the opposite side of the equation

`625z^(2)-150z=-9`

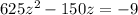
Factor the leading coefficient

`625(z^(2)-(150/625)z)=-9`

`625(z^(2)-(6/25)z)=-9`

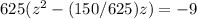
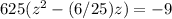
Complete the square. Remember to balance the equation by adding the same constants to each side

`625(z^(2)-(6/25)z+(36/2,500))=-9+(36/4)`

`625(z^(2)-(6/25)z+(36/2,500))=0`

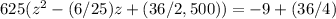
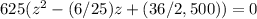
Rewrite as perfect squares

`625(z-6/50)^(2)=0`

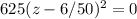
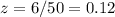`z=6/50=0.12` -----> root with multiplicity 2

so

The area is equal to

`A=625(z-0.12)(z-0.12)=[25(z-0.12)][25(z-0.12)]=(25z-3)^(2)`

The length side of the square is
`b=(25z-3)`

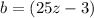
therefore

The perimeter is equal to

`P=4b`

`P=4(25z-3)`

`P=100z-12`

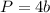
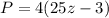
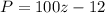
Part 2) Find the perimeter when z = 15 ft.

we have

`P=100z-12`

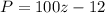
substitute the value of z

`P=100(15)-12=1,488\ ft`