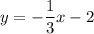Question

Can I get help? the steps too, please. I'd mean a lot to me if someone helped.

Answer 1

`\large\boxed{y=-(1)/(3)x-2}`

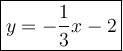
Explanation:

The slope-intercept form of an equation of a line:

`y=mx+b`

m - slope

b - y-intercept

The formula of a slope:

`m=(y_2-y_1)/(x_2-x_1)`

===========================================

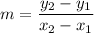
From the graph we have x-intercept (-6, 0) and y-intercept (0, -2) → b = -2.

Calculate the slope:

`m=(-2-0)/(0-(-6))=(-2)/(6)=-(1)/(3)`

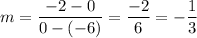
Put the value of the slope m = -1/3 and the value of the y-intercept b = -2 to the equation of a line:

`y=-(1)/(3)x-2`

Answer 2

Remember that the slope intercept formula is:

y = mx + b

m is the slope (
`(rise)/(run)`)

b is the y-intercept

In this case the slope is...

`(-1)/(3)`

^^^This has a negative slope because the line is decreasing from left to right

The y-intercept is...

(0, -2)

so...

y =
`(-1)/(3)`x - 2

Hope this helped!

~Just a girl in love with Shawn Mendes