Answer:
The probability of selecting a heart suit or a face card is
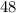 %
%
Step-by-step explanation:
Total number of cards in a deck of cards
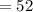
Number of face cards including (4 Kings, 4 Queens and 4 Jacks)
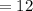
Number of heart cards
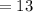
It is case of "OR" probability.
So,
Probability of selecting a Face or heart card
 Probability of selecting a face card
Probability of selecting a face card
 Probability of selecting a heart card
Probability of selecting a heart card
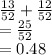
The probability of selecting a heart suit or a face card is
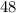 %
%