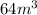Question

Box A has a volume of 512 cubic meters. Box B is similar to box A. To create Box B, Box A's dimensions were divided by 2. What is the volume of Box B?

Answer 1

64 m3 because if u divide it

Answer 2

Answer : The volume of box B is
`64m^3`

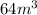
Step-by-step explanation :

Given :

Volume of box A = 512 cubic meter

Let us assumed that the box A and box B are cubes and the dimensions of cube are same i.e, 'a'.

Volume of box A = V =
`a* a* a=a^3`

As per question,

When dimensions of box A divided by 2 then the volume of box B will be:

Volume of box A = V' =
`(a)/(2)* (a)/(2)* (a)/(2)=(a^3)/(8)`

As the box B is similar to box A. So, the volume of box B will be:

Volume of box B = V' =
`(a^3)/(8)`

`V'=(a^3)/(8)=(V)/(8)`

`V'=(512m^3)/(8)=64m^3`

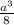
Therefore, the volume of box B is
`64m^3`