Question

A globe company currently manufactures a globe that is 20 inches in diameter. If the dimensions of the globe were reduced by half, what would its volume be? Use 3.14 for π and round your answer to the nearest tenth.

Answer 1

Answer: 523.3 in3

Explanation:

Answer 2

The volume of the reduced globe is
`V=523.3\ in^(3)`

Explanation:

step 1

we know that

The volume of a sphere is equal to

`V=(4)/(3)\pi r^(3)`

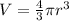
we have

`r=20/2=10\ in` -----> the radius is half the diameter

substitute

The volume of the original globe is

`V=(4)/(3)(3.14)(10)^(3)`

`V=4,186.7\ in^(3)`

step 2

If the dimensions of the globe were reduced by half,

then

The new radio is equal to

`r=10/2=5\ in`

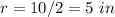
The volume of the reduced globe is

`V=(4)/(3)(3.14)(5)^(3)`

`V=523.3\ in^(3)`

therefore

The volume of the reduced globe is
`V=523.3\ in^(3)`

Compare the volumes

`4,186.7/523.3=8`

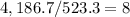
Remember that

If two solids are similar, then the ratio of its volumes is equal to the scale factor elevated to the cube

The scale factor is 1/2

so

`(1)/(2)^(3)=(1)/(8)`

therefore

The original globe is eight times the volume of the reduced globe