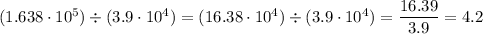Question

I need help on this question cuz sometimes we put the 10 but then sometimes don't i'm confused

Answer 1

4.2

Explanation:

A number in scientific notation consists of a coefficient and a multiplier that is a power of 10.

Terminology and details

The first attachment shows the parts of a number in scientific notation. The coefficient of a number in "scientific notation" is always written with one non-zero digit to the left of the decimal point. (In this form, the number can be said to be "normalized.")

The "coefficient" can also be called the "mantissa" or the "significand," since it is comprised of all of the significant digits of the number.

When these numbers are written by a human, the power of 10 (multiplier) is generally omitted when the exponent is zero. You will notice in the second attachment that the multiplier is included in the "scientific notation" spreadsheet formatting, even when the exponent is zero. Calculators may also include a multiplier with a zero exponent.

Disclaimer: your teacher and/or your curriculum materials may have a different take on omitting ×10^0.

Application

Any spreadsheet and most calculators allow entry and display of numbers in scientific notation. The second attachment shows a spreadsheet result when the numbers are entered in scientific notation. (E is used for ×10^ by these devices.)

The third attachment shows a calculator result when formatted for scientific notation (first line) and for normal output presentation (remaining lines).

Using the above-described requirement for a non-zero exponent in a number in scientific notation, we can write your result as ...

`(1.638\cdot10^5)/(3.9\cdot10^4)=\boxed{4.2}`

__

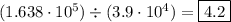
Additional comment

The mantissa (m) in a number in scientific notation must be in the range ...

1 ≤ m < 10

We note that the result of dividing the mantissas in the given problem will be ...

1.638 ÷ 3.9 = 0.42 < 1

Straightforward application of the rules of exponents would give the result as ...

`(1.638\cdot10^5)/(3.9\cdot10^4)=(1.638)/(3.9)\cdot10^(5-4)=0.42\cdot10^1`

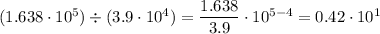
This requires an extra step to normalize the number to 4.2×10^0 = 4.2.

We can avoid the normalization step at the end by denormalizing one of the numbers at the beginning:

`(1.638\cdot10^5)/(3.9\cdot10^4)=(16.38\cdot10^4)/(3.9\cdot10^4)=(16.39)/(3.9)=4.2`