Answer:
The equation for the axis of symmetry is x=1.5.
Explanation:
It is given that a quadratic function passes through the points ( − 5 , − 8 ) and ( 8 , − 8 ) .
In the given points y-coordinates are same, i.e., -8. It means both the points lie on the horizontal line y=-8.
If a quadratic function passes through two points (a,c) and (b,c), then the equation for the axis of symmetry is

According to the given points a=-5, b=8 and c=-8. Put these value in the above formula.
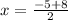
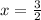

Therefore the equation for the axis of symmetry is x=1.5.