Answer: 7.94
Explanation:
The formula to calculate the standard deviation for binomial distribution :-
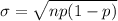 , where n is the number total of trials and p is the probability of getting success in each trial.
, where n is the number total of trials and p is the probability of getting success in each trial.
Given : The probability of the population has a particular genetic mutation=0.1
If 700 people are randomly selected, then the standard deviation for the number of people with the genetic mutation in such groups of 700 will be :-

Hence, the standard deviation for the number of people with the genetic mutation in such groups of 700 = 7.94