Answer:
(a)2.7 m/s
(b) 5.52 m/s
Step-by-step explanation:
The total of the system would be conserved as no external force is acting on it.
Initial momentum = final momentum
⇒(4.30 g × 943 m/s) + (730 g × 0) = (4.30 g × 484 m/s) + (730 g × v)
⇒ 730 ×v = (4054.9 - 2081.2) =1973.7
⇒v=2.7 m/s
Thus, the resulting speed of the block is 2.7 m/s.
(b) since, the momentum is conserved, the speed of the bullet-block center of mass would be constant.
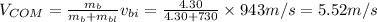
Thus, the speed of the bullet-block center of mass is 5.52 m/s.