Answer:
The fundamental set of solutions are:
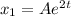
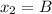


Hence, the fundamental set of solutions are:
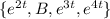
Explanation:
We are given a set as:
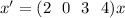
which in matrix form could be given by:
![\left[\begin{array}{ccc}x_1'\\x_2'\\x_3'\\x_4'\end{array}\right]=[2\ \ 0\ \ 3\ \ 4]\left[\begin{array}{ccc}x_1\\x_2\\x_3\\x_4\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/k5ml53k8nc26m9zvk145emgs8lfdvscftl.png)
Now, this could also be written in the form:
![\left[\begin{array}{ccc}x_1'\\x_2'\\x_3'\\x_4'\end{array}\right]=\left[\begin{array}{ccc}2\cdot x_1\\0\cdot x_2\\3\cdot x_3\\4\cdot x_4\end{array}\right]\\\\i.e.\\\\\left[\begin{array}{ccc}x_1'\\x_2'\\x_3'\\x_4'\end{array}\right]=\left[\begin{array}{ccc}2x_1\\0\\3x_3\\4x_4\end{array}\right]](https://img.qammunity.org/2020/formulas/mathematics/college/d6vnvcytrl6mly9n2opgdwcz80auuk46wk.png)
Now, in order to find each of the x_i's we have:

where A is a constant.
Similarly we have:

where B is a constant.
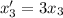
As done for x_1 we have:

and
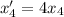
Hence,
