Question

Each cone of the hourglass has a height of 15 millimeters. The total height of the sand within the top portion of the hourglass is 45 millimeters. The radius of both cylinder and cone is 6 millimeters. Sand drips from the top of the hourglass to the bottom at a rate of 10π cubic millimeters per second. How many seconds will it take until all of the sand has dripped to the bottom of the hourglass?

Answer 1

The answer is 126 seconds.

Explanation:

Volume of the cone is given as:

`(1)/(3) \pi r^(2) h`

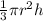
Volume of cylinder is given as:

`\pi r^(2) h`

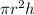
We can find the total volume of the sand by adding volume of cone and volume of cylinder.

Height for cylinder is 30 mm (as we will subtract the cone height from 45mm that is 45-15=30)

Total volume of sand =
`\pi /3*(6^(2))*(15)` +
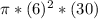`\pi *(6)^(2)*(30)` = 1260π mm³

Given is - Sand drips from the top of the hourglass to the bottom at a rate of 10π cubic millimeters per second.

So, for all the sand to drip down, it will take
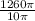`(1260\pi )/(10\pi )`= 126 seconds.