Question

St has endpoint S(-2,-4) and T(6,8). Find the coordinates of the midpoint of ST

Answer 1

`\large\boxed{(2,2)}`

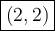
Explanation:

In this question, we're trying to find the midpoint of the line segment "ST"

In order to find the midpoint, we're going to need to sue the midpoint formula.

Mid point formula:

`m=({(x1+x2)/(2)},(y1+y2)/(2))`

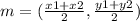
You would plug the numbers into the right spot. Plugging the first "x" coordinate to x1 and etc.

Your equation should look like this:

`m=({(-2+6)/(2)},(-4+8)/(2))`

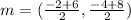
Now you will solve:

`m=({(-2+6)/(2)},(-4+8)/(2))\\\\\text{Lets add all the numbers}\\\\m=({(4)/(2)},(4)/(2))\\\\\text{Now, you would simply divide the fractions}\\\\m=(2,2)`

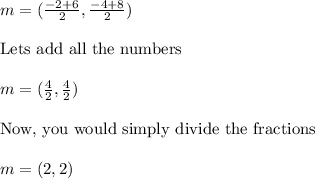
When you're done solving, you should get (2,2)

This means that the midpoint of ST is (2,2)

I hope this helped you out.

Good luck on your academics.

Have a fantastic day!

Answer 2

The formula for midpoint is (
`(x_(1)+x_(2))/(2)`,
`(y_(1)+y_(2))/(2)`)

In this case:

`x_(1) =-2\\x_(2) =6\\y_(1) =-4\\y_(2) =8`

^^^Plug in these number into the formula given above...

(
`(-2 + 6)/(2)`,
`(-4 + 8)/(2)`)

(
`(4)/(2)`,
`(4)/(2)`)

Simplify the fractions:

(2, 2)

^^^This is the coordinate of the midpoint

Hope this helped!

~Just a girl in love with Shawn Mendes