Question

A bullet of mass 0.093 kg traveling horizontally at a speed of 100 m/s embeds itself in a block of mass 2.5 kg that is sitting at rest on a nearly frictionless surface. (a) What is the speed of the block after the bullet embeds itself in the block?

Answer 1

Step-by-step explanation:

It is given that,

Mass of the bullet, m₁ = 0.093 kg

Initial speed of bullet, u₁ = 100 m/s

Mass of block, m₂ = 2.5 kg

Initial speed of block, u₂ = 0

We need to find the speed of the block after the bullet embeds itself in the block. Let it is given by V. On applying the conservation of linear momentum as :

`m_1u_1+m_2u_2=(m_1+m_2)V`

`V=(m_1u_1+m_2u_2)/((m_1+m_2))`

`V=(0.093\ kg* 100\ m/s+0)/((0.093\ kg+2.5\ kg))`

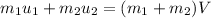
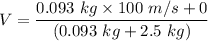
V = 3.58 m/s

So, the speed of the bullet is 3.58 m/s. Hence, this is the required solution.