Question

Light passes through a lens with a refractive index of 1.6, and front and back sur faces with radii of 11 and 15 cm, respectively. a. What is the focal length of the lens? b. What would be the focal length of an identically proportioned lens 100 times larger?

Answer 1

a).f =68.75 cm

b). focal length will be 100 times larger.

Step-by-step explanation:

Given :

Refractive index of the lens, n = 1.6

Front surface radius,
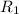`R_(1)` = 11 cm

Back surface radius,
`R_(2)` = 15 cm

Therefore we know for a focal length that

a). Focal length,
`(1)/(f) = (n-1)\left [ (1)/(R_(1)) -(1)/(R_(2))\right ]`

`(1)/(f) = (1.6-1)\left [ (1)/(11) -(1)/(15)\right ]`

`(1)/(f) = (0.6)* \left [ (15-11)/(15* 11) \right ]`

f =68.75 cm

b). We know that aperture and focal length of lens are related directly. If aperture is small, focal length is small and when aperture is large , the focal length is more.

Thus, when the aperture is 100 times larger, the focal length will also be 100 times larger.