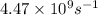Answer : The frequency of a photon of radiation is,
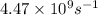
Explanation : Given,
Wavelength of the radiation = 670.8 nm
First we have to convert wavelength form 'nm' to 'm'.
Conversion used :
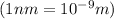
So, the wavelength of the radiation = 670.8 nm =
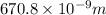
Now we have to calculate the frequency of a photon of radiation.
Formula used :
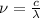
where,
 = frequency of a photon of radiation
= frequency of a photon of radiation
 = wavelength of the radiation
= wavelength of the radiation
c = speed of light =
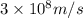
Now put all the given values in the above formula, we get the frequency of a photon of radiation.

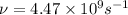
Therefore, the frequency of a photon of radiation is,