Answer:
A) 1.2
B) 1.1
Step-by-step explanation:
A)
F = force required to start the box moving = 36.0 N
m = mass of the box = 3 kg
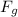 = weight of the box = mg = 3 x 9.8 = 29.4 N
= weight of the box = mg = 3 x 9.8 = 29.4 N
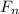 = normal force acting on the box by the floor
= normal force acting on the box by the floor
normal force acting on the box by the floor is given as
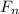 =
=
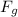 = 29.4
= 29.4
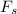 = Static frictional force = F = 36.0 N
= Static frictional force = F = 36.0 N
 = Coefficient of static friction
= Coefficient of static friction
Static frictional force is given as
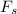 =
=

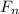
36.0 =
 (29.4)
(29.4)
 = 1.2
= 1.2
B)
a = acceleration of the box = 0.54 m/s²
F = force applied = 36.0 N
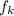 = kinetic frictional force
= kinetic frictional force
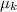 = Coefficient of kinetic friction
= Coefficient of kinetic friction
force equation for the motion of the box is given as
F -
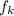 = ma
= ma
36.0 -
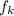 = (3) (0.54)
= (3) (0.54)
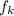 = 34.38 N
= 34.38 N
Coefficient of kinetic friction is given as
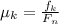
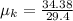
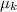 = 1.1
= 1.1