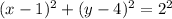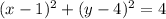Question

A circle has its center at (1, 4) and a radius of 2 units. What is the equation of the circle? (1 point) (x + 2)2 + (y + 4)2 = 2 (x − 1)2 + (y − 4)2 = 4 (x + 1)2 + (y − 4)2 = 4 (x − 1)2 + (y − 4)2 = 2

Answer 1

3rd one. The general form of a circle is set equal to the radius squared. So right side is 4 then plug in values until true.

Answer 2

The answer is the second option

`(x-1)^(2)+(y-4)^(2)= 4`

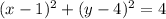
Explanation:

The general equation of a circle is:

`(x-h)^(2)+(y-k)^(2)= r^(2)`

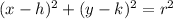
in this equation (h,k) is the center of the circle and r is the radius, so if the center is in (1,4) and the radius is 2, the values of the constants are:

h = 1

k = 4

r = 2

And the formula for this circle is:

`(x-1)^(2)+(y-4)^(2)= 2^(2)`

`(x-1)^(2)+(y-4)^(2)= 4`