Question

What is the total surface area of the rectangular pyramid below

Answer 1

Total surface area of the pyramid = 225 ft²

Explanation:

Total surface area of the given pyramid is defined by

(Area of rectangular base) + 2(area of two triangular sides with height 8 ft and base 12 ft) + 2(area of two triangles with height 9.5 ft and base 6 ft)

Total surface area = (12×6) + 2[
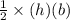`(1)/(2)*(h)(b)`]+2[
`(1)/(2)*(h')(b')`]

= 72 + 2[
`(1)/(2)*(8)(12)`]+2[
`(1)/(2)*(9.5)(6)`]

= 72 + 96 + 57

= 225 ft²

Therefore, total surface area of the pyramid is 225 ft²

Answer 2

S.A. = 225 ft²

Explanation:

We have

the rectangle 12ft × 6ft

two triangles with the base b = 12ft and the height h = 8ft

two triangles with the base b = 6ft and the height h = 9.5ft.

The formula of an area of a rectangle l × w:

`A=lw`

Substitute:

`A_1=(12)(6)=72\ dt^2`

The formula of an area of a triangle:

`A=(bh)/(2)`

Substitute:

`A_2=((12)(8))/(2)=48\ ft^2`

`A_3=((6)(9.5))/(2)=28.5\ ft^2`

The Surface Area:

`S.A.=A_1+2A_2+2A_3`

Substitute:

`S.A.=72+2(48)+2(28.5)=225\ ft^2`