Question

The following set of coordinates most specifically represents which figure? (−5, 6), (−1, 8), (3, 6), (−1, 4) (6 points) Parallelogram Rectangle Rhombus Square

Answer 1

answer is Rhombus

Explanation:

Answer 2

Rhombus

Explanation:

The given points are A(−5, 6), B(−1, 8), C(3, 6), D(−1, 4).

We use the distance formula to find the length of AB.

`|AB|=√((-1--5)^2+(8-6)^2)`

`|AB|=√(16+4)`

`|AB|=√(20)`

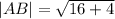
The length of AD is

`|AD|=√((-1--5)^2+(6-4)^2)`

`|AD|=√(16+4)`

`|AD|=√(20)`

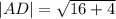
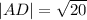
The length of BC is:

`|BC|=√((-1-3)^2+(8-6)^2)`

`|BC|=√(16+4)`

`|BC|=√(20)`

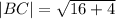
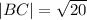
The length of CD is

`|CD|=√((-1-3)^2+(6-4)^2)`

`|CD|=√(16+4)`

`|CD|=√(20)`

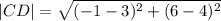
Since all sides are congruent the quadrilateral could be a rhombus or a square.

Slope of AB
`=(8-6)/(-1--5)=(1)/(2)`

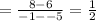
Slope of BC
`=(8-6)/(-1-3)=-(1)/(2)`

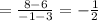
Since the slopes of the adjacent sides are not negative reciprocals of each other, the quadrilateral cannot be a square. It is a rhombus