Question

Can someone please help me, I need to know the missing side length (x) using trigonometric ratios.

Answer 1

x=6/cos(72) is the exact answer.

`x \approx 19.416`.

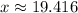Explanation:

Sine

opposite

hypotenuse

Cosine

adjacent

hypotenuse

Tangent

opposite

adjacent

Soh Cah Toa is an abbreviation used to help remember the trigonometric ratios.

We see we have the angle measurement 72 degrees given at the bottom. I'm going to label my sides with respect to that angle.

The hypotenuse is x.

The adjacent is 6.

We don't have anything about the opposite side but we don't need it to find x.

So anyways we have this:

The hypotenuse is x and

the adjacent is 6.

If you look at the ratios we want to use cosine.

`\cos(72)=(6)/(x)`

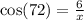
or

`(\cos(72))/(1)=(6)/(x)`

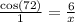
We are going to cross multiply.

x cos(72)=1 (6)

cos(72) * x=6

Now we are going to divide both sides by cos(72) giving us:

x=6/cos(72)

x=6/cos(72) is the exact answer.

Let's put it into our calculator now:

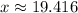`x \approx 19.416`.