Question

The maximum value of 12 sin 0-9 sin²0 is: -​

Answer 1

4

Explanation:

The question is not clear. You have indicated the original function as 12sin(0) - 9sin²(0)

If so, the solution is trivial. At 0, sin(0) is 0 so the solution is 0

However, I will assume you meant the angle to be
`\theta` rather than 0 which makes sense and proceed accordingly

We can find the maximum or minimum of any function by finding the first derivate and setting it equal to 0

The original function is

`f(\theta) = 12sin(\theta) - 9 sin^2(\theta)`

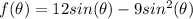
Taking the first derivative of this with respect to
`\theta` and setting it equal to 0 lets us solve for the maximum (or minimum) value

The first derivative of
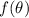`f(\theta)` w.r.t
`\theta` is

`12\cos\left(\theta\right)-18\cos\left(\theta\right)\sin\left(\theta\right)`

And setting this = 0 gives

`12\cos\left(\theta\right)-18\cos\left(\theta\right)\sin\left(\theta\right) = 0`

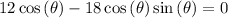
Eliminating
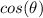`cos(\theta)` on both sides and solving for
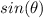`sin(\theta)` gives us

`sin(\theta) = (12)/(18) = (2)/(3)`

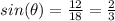
Plugging this value of
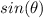`sin(\theta)` into the original equation gives us

`12((2)/(3)) - 9((4)/(9) ) = 8 - 4 = 4`

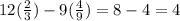
This is the maximum value that the function can acquire. The attached graph shows this as correct