Question

The terminal velocity is not dependent on which one of the following properties? the drag coefficient 1 the force of gravity 2 cross-sectional area 3 air density 4 the falling time 5 terminal velocity depends on all of the 6 given parameters

Answer 1

Answer: the falling time

Step-by-step explanation:

When a body or object falls, basically two forces act on it:

1. The force of air friction, also called "drag force"
`D`:

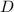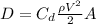`D={C}_(d)(\rho V^(2) )/(2)A` (1)

Where:

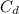`C_ {d}` is the drag coefficient

`\rho` is the density of the fluid (air for example)

`V` is the velocity

`A` is the transversal area of the object

So, this force is proportional to the transversal area of ​​the falling element and to the square of the velocity.

2. Its weight due to the gravity force
`W`:

`W=m.g`

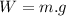
(2)

Where:

`m` is the mass of the object

`g` is the acceleration due gravity

So, at the moment when the drag force equals the gravity force, the object will have its terminal velocity:

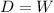`D=W` (3)

`{C}_(d)(\rho V^(2) )/(2)A=m.g` (4)

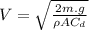`V=\sqrt{\frac{2m.g}{\rho A{C}_(d)}}` (5) This is the terminal velocity

As we can see, there is no "falling time" in this equation.

Therefore, the terminal velocity is not dependent on the falling time.