Question

The equation 2x^2 – 8x = 5 is rewritten in the form of 2(x – p)^2 + q = 0. what is the value of q?

Answer 1

q = -13

Explanation:

The given equation is:

`2x^(2)-8x=5`

Taking 2 as common from left hand side, we get:

`2(x^(2)-4x)=5\\\\2[x^(2) - 2(x)(2)]=5`

The square of difference is written as:

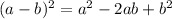`(a-b)^(2)=a^2 - 2ab + b^(2)` Equation 1

If we compare the given equation from previous step to formula in Equation 1, we note that we have square of first term(x), twice the product of 1st term(x) and second term(2) and the square of second term(2) is missing. So in order to complete the square we need to add and subtract square of 2 to right hand side. i.e.

`2[x^(2)-2(x)(2)+(2)^2-(2)^(2)]=5\\\\ 2[x^(2)-2(x)(2)+(2)^2]-2(2)^(2)=5\\\\ 2(x-2)^(2)-2(4)=5\\\\ 2(x-2)^2-8=5\\\\ 2(x-2)^(2)-8-5=0\\\\ 2(x-2)^(2)-13=0`

Comparing the above equation with the given equation:

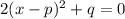`2(x-p)^(2)+q=0`, we can say:

p = 2 and q= -13