Answer:
Interpretation: The zeros are where the daily profit is $0.00.
Zeroes are x = 1.58 and x = 4.41.
Explanation:
Given function,

For finding the zeros,
y = 0,

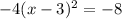
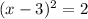
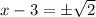
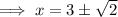
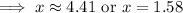
Hence, the zeroes of the function are x = 1.58 and x = 4.41,
∵ x represents the price of a taco and y represents daily profit,
Therefore, the zeroes are where the daily profit is $ 0.00.