Question

Find the following standard normal probability: p(-1.23 szs +1.23) OA..7814 B. .8907 O C..1093 D. .2186

In the formula used to convert "real-world" data values into z numbers, the standard deviation of the data is never considered. O True O False

Answer 1

Answer: a) 0.7814

b) False

Explanation:

To find : The p-value of z , where

`-1.23<z< +1.23`

`P(-1.23<z< +1.23)=1-P(z<-1.23)\\\\=1-0.1093=0.7814`

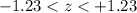
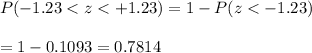
Hence, the standard normal probability
`P(-1.23<z< +1.23)=0.7814`

In the formula used to convert "real-world" data values into z numbers, the standard deviation of the data is considered.

The formula to calculate the z score is
`z=(x-\mu)/(\sigma)`.

If sample size (n) is given then , the
`z=(x-\mu)/((\sigma)/(√(n)))`

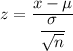
Hence, the statement is false.