Question

For the month of June in a certain​ city, 41​% of the days are cloudy. Also in the month of June in the same​ city, 21​% of the days are cloudy and rainy. What is the probability that a randomly selected day in June will be rainy if it is cloudy​?

Answer 1

0.5122 or 51.22%

Explanation:

In a certain city, in June Probability of cloudy days = P(cloudy) = 0.41

Probability of cloudy and rainy = P(cloudy and rainy) = 0.21

Probability of rainy if we already know it is cloudy =
`\frac{\text{[P(cloud and rainy)]}}{[P(cloud)]}`

=
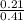`(0.21)/(0.41)` = 0.512195122 ≈ 0.5122

Therefore, the probability that a randomly selected day in June will be rainy if it is cloudy is 0.5122 or 51.22%