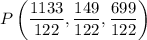Answer:
The point of intersection
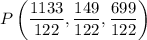
Explanation:
Equation of line:
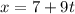
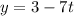
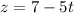
Equation of plane:

We need to find the point of intersection of line and plane.
Point of intersection: When both line and plane meet at single point.
So, put the value of x, y and z into plane.
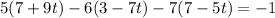
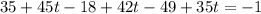

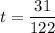
Substitute the value of t into x, y and z
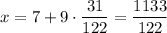
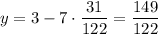
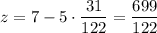
Point of intersection:

Hence, The point of intersection